The T-shirt equivalent of Brien Taylor

Today I witnessed the wearing by a stranger of this shirt [drag your cursor over the picture and click on the closeup of the graphic]. Like Brien Taylor, upon first glance the article of clothing was filled with promise. The Yankees' first overall pick never made it to the majors in part because of an injury sustained in a barfight. How could a t-shirt match this disappointment?

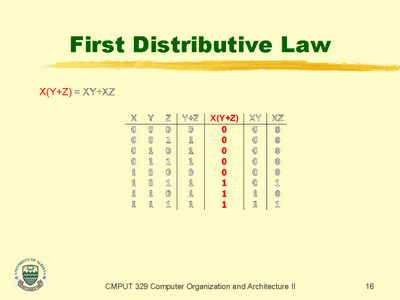
(1) I'm the wrong person to assess this sort of thing, but it might be true that the FOIL method sticks with people more than most things uttered by secondary school math instructors. If true, I accept this as an argument in favor of teaching FOIL. Nevertheless, the FOIL method is pedagogically bankrupt. The proper name for the rule by which one multiplies a sum (a₁ + ... + a_n) by another sum (b₁ + ... + b_n) is (possibly many applications of) the distributive law. The distributive law says a(b+c)=ab+ac. Applying it twices gives the equation (a+b)(c+d)=a(c+d)+b(c+d)=ac+ad+bc+bd. Teaching the FOIL method is bad because it replaces with a trick the axiom which relates the operations of addition and multiplication.

If most people would answer the question “Why does FOIL work?” by mentioning the distributive law, I would be happier. But not only is this unlikely, once a student understands how the distributive law allows one to find an expression equivalent to (a+b)(c+d), the trick is unnecessary.

Finally, the FOIL method singles out the multiplication of two binomials. An expert FOILer might be at a loss to find an expression equivalent to (a+b+c)(d+e+f), say. (You could FOIL twice, but then you SO have a crush on the distributive law it's, like, ridiculous.) But it's clear how to proceed if multiplication of binomials it taught by the distributive law; likewise with expressions involving more than one multiplication symbol.

In fact people like to study sets with addition and multiplication operations which are related by a distributive law, and emphasizing the distributive law gives context that FOIL does not.



(2) Even if you like the FOIL method, which you shouldn't, why would you glorify its application to the multiplication (a+b)(a+b)?! This might give the reader the misleading impression that FOIL is only good for simplifying (a+b)², when in fact it works for (a+b)(c+d). Is it possible that not only does the shirt's designer know how to multiply only binomials, (s)he knows only how to square a binomial?! Eek.

Also (this is a minor point), once one chooses to simplify (a+b)², it's irresistible to write a² + 2ab + b² in the stead of a² + ab + ba + b². In arbitrary rings multiplication need not be commutative (e.g. multiplication of matrices is not), yet the distributive law (and therefore FOIL) must hold. Writing (a+b)(c+d)=ac+ad+bc+bd gives one less of a chance to assume multiplication is commutative.

This is “the perfect shirt to wear to your algebra tests” until c+d is distinct from a+b. So the knowledge on this shirt should be good for an F, which by the way is the grade earned by this shirt in the Execution category.

(3) (a+b)(a+b) is not an equation. It's an expression. An equation asserts that two expressions are equal. The equation the FOIL method asserts is (a+b)(a+b)=a² + ab + ba + b² (=a² + 2ab + b² if the ring is commutative). Maker of this shirt, you didn't have many words to choose. You should have chosen the right ones.

(4) In your description, you are missing some commas. The period doesn't belong inside the parentheses. By what gimmicks did you learn grammar?